One of the things one realises when one studies the more advanced parts of maths is that you can prove very obvious things, but the proof requires pretty advanced technical knowledge. Thus, paradoxically, the simpler and more "obvious" the proposition, the harder it is to prove.
The example below is an exercise to prove what may be the most common sensical proposition: the shortest path between two points is a straight line.
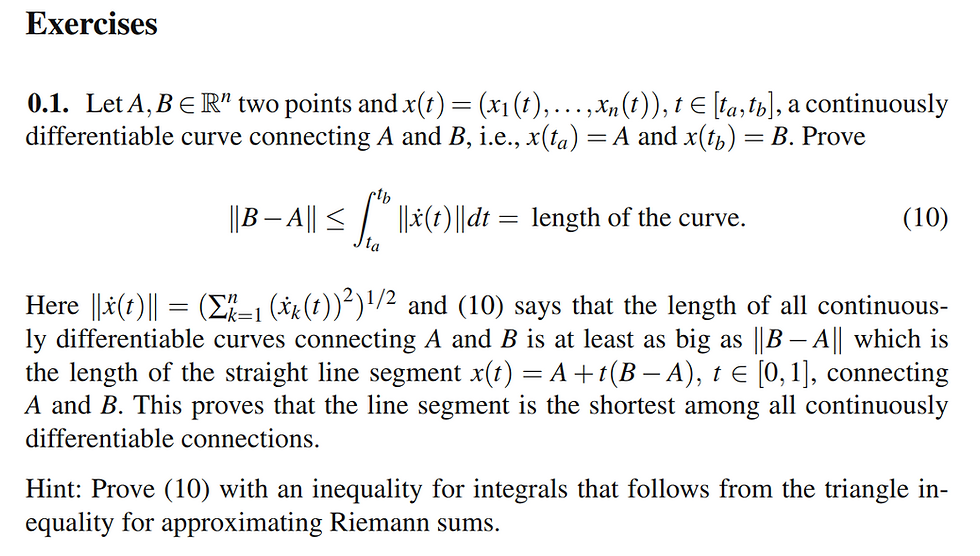
One will need a fair bit of knowledge of calculus and vectors to prove this theorem. Speculatively, why it is difficult to prove a theorem as simple as this is that you will need to master even more abstract and elementary things, which is normally beyond the standard experience or comprehension of most people.
That said, the reality is that most of us go through life assured of this simple proposition without the need to master a battery of calculus or vector concepts.
I suggest that the same principle applies to the divine existence. It is such a properly basic and obvious proposition that no one needs to be able to prove it. But because it is such a basic proposition, if one tried to prove it one will need to master a battery of abstract elementary concepts which transcends the normal ken of experience.
As such, I suggest that this is a good analogy for my via media approach towards the epistemology of divine existence. We can, and indeed should, treat it as properly basic and obviously to be presupposed, and at the same time that it can be demonstrated, but the proof is hard. Fortunately, most of us can go through life assuming basic maths propositions without mastering the calculus of variations.